Case Study Analysis of Database Construction for Smoke Control Simulation Experiments Using Scale Model
Article information
Abstract
Most studies on fire properties, including smoke control, have been based on simulations. However, the results of simulation experiments have an average error rate of approximately 36.7%, and data with low reliability are obtained, compared to theoretical values and actual-scale measurements. In addition, experimental studies using scale models exhibit an average error rate of 6.9%, significantly lower than those of simulation experiments. In this study, a method for decreasing the error rate of simulation experiments was investigated by constructing a database using scale-model smoke control experiments.
1. Introduction
As the sizes of buildings increase owing to the rapid industrial development and advanced construction technology of modern society, emphasis on human safety is increasing [1]. Accordingly, studies on safety, including firefighting equipment, fire-resistant materials, and smoke control, are required.
Table 1 lists the statistics of fire accident deaths over the last five years reported by the National Fire Agency. Among the fire accident deaths, suffocation deaths caused by smoke and toxic gas inhalation represented more than 35.0%. The death rate reached 57.7% when smoke and toxic gases were accompanied by burns [2]. In this regard, identifying the characteristics of smoke has been emphasized, and studies on smoke flow and smoke control have been conducted. However, performing actual-scale smoke control experiments involves high costs and risk [3]. In most cases, computer simulations are used to identify the flow characteristics of smoke because of the nature of smoke control experiments that require the reproduction of buildings and setting of various conditions. Computer simulation is convenient, but the sufficient reliability of the data cannot be guaranteed. Kim et al. [4] compared Fire Dynamics Simulator (FDS) data with actual-scale experimental data obtained for a fire experiment. They reported that the error range increased, and low reliability was observed owing to insufficient data used in the simulation.
For scale-model experiments, data with higher reliability than those of simulation experiments can be obtained. Kim et al. [5] conducted a model experiment at a scale of 1:20 and assumed a fire inside a tunnel. They reported that the results of the experiment are applicable to actual tunnel designs because the experimental and numerical analysis results showed an error range lower than 10%. Scale-model experiments have been performed using limited models such as tunnels, and various types of materials are required to combine steel beams, acrylic materials, steel plates, cerakwool, and other materials [6].
Scale-model experiments are valuable methods for supplementing the error rate and improving the reliability of simulation results. Consistent data can be obtained when experiments on fire properties are conducted in actual space, but scale-model experiments enable a more detailed investigation than experiments in actual space through relatively convenient designs and preparation. Scale-model experiments minimize the error rate and validate the errors attributed to insufficient databases.
Performance-based design, which is an engineering-based approach to fire safety performance evaluation, has been implemented to overcome the limitations of existing uniform specification-based design methods. The fire safety performance of new buildings has been assessed using simulation software for performance-based design. Despite its convenience, simulations have disadvantages, such as inappropriate case selection, low reliability, and the absence of quantitative criteria for simulation input values [7,8]. Therefore, this study attempted to analyze the applicability of experiments on fire properties and smoke control by constructing a database for simulation experiments using scale-model experiments.
2. Research Trends and Error Rate Analysis Method
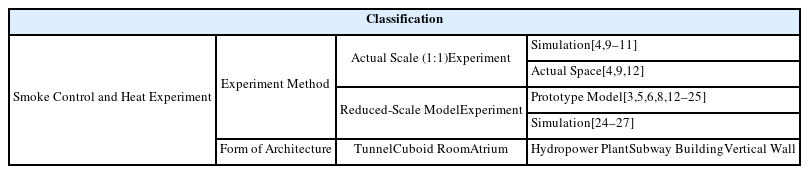
Table 2 lists the analysis of previous studies on fire. In these studies, experiments were conducted on various types of buildings. These studies used a 1:1 scale and reduced scales. For the 1:1 scale, experiments were conducted using actual space or simulations; for the reduced scales, models and simulations were used.
2.1 Analysis of previous studies on fire properties
For studies that used scale models, the minimum scale was 1:2.5, and the maximum scale was 1:50. The types of buildings analyzed for the scale-model experiments included tunnels [3,5,13,14,17-22,27], underground railway stations [6], parking lots [12], hydroelectric power plants [26], atrium [16], and cuboid rooms [23]. Most of the experiments were performed using tunnel-type models. This is possibly because it is easier to prepare tunnel-type models than other model types.
Previous simulation studies were based on scales ranging from 1:1 to 1:3. Most of these simulation studies were conducted in cuboid rooms [5], whereas some were conducted on vertical walls [8] and wood stacks [9]. For scale-model experiments, the temperature distribution and smoke flow were the main analyzed properties. In the case of the simulation studies, various data such as the heat release rate, heat flux, and CO, CO₂, and O₂ concentrations were analyzed.
Figure 1 shows an example of a smoke control experiment, Figure 2 shows an example of a simulation experiment with FDS.
2.2 Error rate analysis method
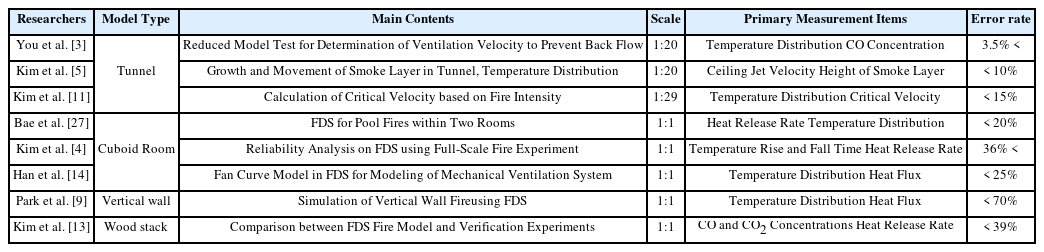
Table 3 lists a summary of the smoke control and heat experiments performed on actual scales, reduced models, and simulations. Most of the experiments were based on the tunnel type because the model construction was relatively convenient. For the tunnel-type model, experiments were conducted at a minimum ratio of 1:4 [27] and a maximum ratio of 1:40 [14]. In the case of the cuboid-type model, experiments were performed at a minimum ratio of 1:6 [15]. The most reduced experiment was performed for the atrium-type architecture with a ratio of 1:50 [16].
Eq. (1) was used to calculate the error rates in previous studies (Table 3). The measured values were obtained using the "Graph Data Extractor" software.
The theoretical value is the actual-scale experimental value or the theoretically calculated value, whereas the measured value is the value obtained when a scale model or simulation was used.
3. Analysis Results
3.1 Error rate analysis results of previous studies
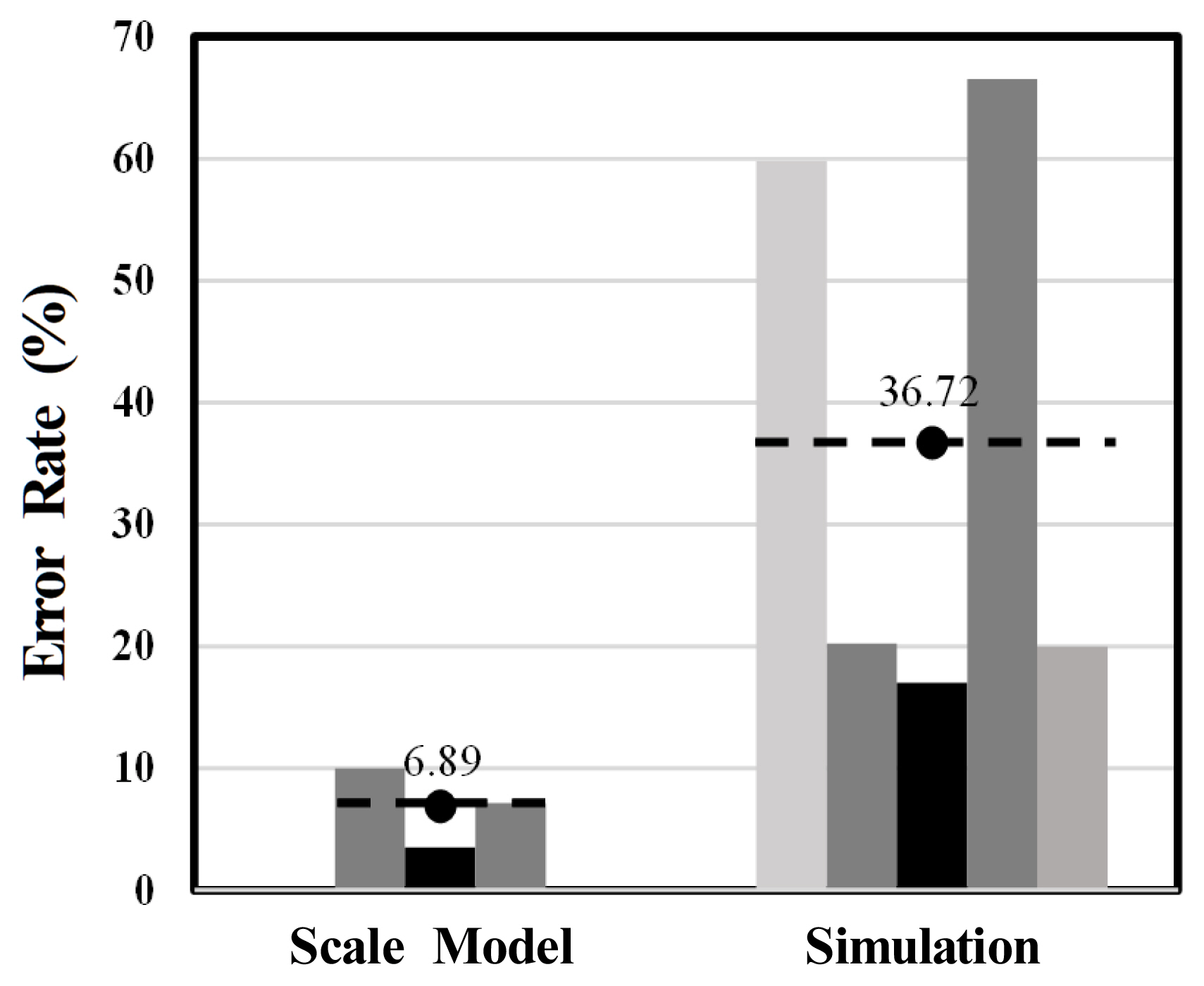
Figure 3 shows the error rates and average values of the experiments for which the error rates are calculated among the experiments listed in Table 3. The error rate was calculated by comparing the results of the scale-model experiments with the theoretical and actual-scale experimental results, and the same method was used for the simulation experiments. A comparison of the two experimental groups showed that the scale-model experiments yielded minimum and maximum error rates of 1.5% and 14.4%, respectively, whereas the simulation experiments yielded minimum and maximum error rates of 3.6% and 116.0%, respectively. The average error rates for the scale-model and simulation experiments were 6.9% and 36.7%, respectively. The scale-model experiments generally yielded lower error rates than the simulation experiments.
3.2 Results of analyzing formulas used in smoke control experiments
Table 4 lists the formulas used in previous scale-model experiments. Governing equations and the Froude scaling law were the most frequently used formulas in the studies. Among the governing equations, four types of continuity equations (mass conservation) and four types of momentum equations (momentum conservation) were used for the calculations. Scalar equations were expressed in two ways, and the equation of state was expressed in one way.
In the Froude scaling law, the heat release rate, velocity ratio, and time ratio were expressed as length-related equations. Although various expression methods are available, only one interpretation was expressed.
When the governing equations and Froude scaling law were considered, the widely used equations were as follows.
Scale-model experiments are performed by reducing the prototype building to a specified ratio. Therefore, compensation is determined according to the ratio using the Froude Scaling law to apply data to the prototype building:
where Q is the heat release rate, V is the flow velocity, L is the length, M is the model, P is the prototype, and α is the length ratio. The analysis of Eqs. (2) and (3) showed that the velocity ratio between the model and prototype was proportional to the square root of the length ratio. The time ratio was also proportional to the square root of the length because it is related to the velocity ratio. However, according to Eq. (4), the heat release rate was proportional to the length ratio to the power of 5/2.
The smoke analysis is governed by equations for density, mass, momentum, and scalar quantities. The governing equations used in previous experiments can be expressed as follows:
• Continuity equation
• Momentum equation
• Scalar equation
• Equation of state
where μ is the viscosity coefficient, μeff is the effective viscosity coefficient, and Γ eff is the effective diffusion coefficient. ø represents the temperature, smoke concentration, and velocity components. P is the pressure, ρ is the density, R is the specific gas constant, and T is the temperature. Subscripts i, j, and k indicate the x-, y-, and z-directions in the rectangular coordinate system, respectively.
4. Conclusion
In this study, a method for preparing a scale model and applying it to smoke control experiments was examined. The following conclusions were drawn.
1) Previous studies on smoke control analysis using simulations showed an average error rate of 36.7% and exhibited low reliability owing to insufficient databases. In contrast, scale-model experiments tended to exhibit higher reliability than simulation experiments, with an average error rate of 3.6%.
2) The equations used in previous studies on smoke control were governing equations and the Froude scaling law. If Eqs. (2)-(9) mentioned above are used in future scale-model and smoke control experimental research, it will be possible to obtain the error rate by calculating the theoretical value and comparing it with the measured value or simulation result. The equations are expected to help in constructing a more accurate database.
3) National laws and standards related to smoke control systems are insufficient compared to those of other countries. If data with lower error rates are applied to simulations using scale-model experiments based on the results of this study, it will be possible to assess the safety of buildings with higher reliability.
Acknowledgments
This work was supported by the National Research Foundation of Korea (NRF) grant funded by the Korea government (MSIT) (No.2022R1F1A1073333).
Notes
Conflicts of Interest
The authors declare no conflict of interest.
Author Contributions
Conceptualization, W.K. and T.L.; methodology, W.K.; software, W.K and K.J..; validation, W.K. and T.L.; formal analysis, H.I.; investigation, H.I.; resources, W.K.; data curation, H.I.; writing—original draft preparation, H.I.; writing—review and editing, W.K.; visualization, T.L.; supervision, W.K.; project administration, T.L.; funding acquisition, K.J. All authors have read and agreed to the published version of the manuscript.